by Patrick and Gael Flanagan

Remember when you were a child, how you marveled at the way water made a vortex when it went down the drain? The vortex is a basic law of the universe. We shall see that the vortex is present from the interstellar nebulae to the atom itself. The energy of the vortex molds the Universe from the microcosm to the macrocosm. It is the formative energy of creation.

Every organ in every living thing is a part of a vortex. All organs are literally vortex formed. Dr. Theodore Schwenk of Weleda Laboratories has published an excellent book entitled the Sensitive Chaos in which he gives example after example of the vortexial formative process in nature. The primordial force involved in vortex formation is tuned to the woof and warp of the Universal matrix.

All flowing fluids, although appearing to be uniform in structure are divided into extensive inner surfaces. When any discontinuity appears in the fluid, such as an obstruction, the inner surfaces flow at different rates and form themselves into a spiral or vortex. When a vortex is formed it appears to have a body of its own. It separates from the rest of the fluid and behaves as if it were a solid mass. It is self contained yet bound up with the whole.

We find that the vortex has a rhythm of its own. It shrinks in diameter and increases in length at one moment, at the next it expands in diameter and shrinks in length. It continues this oscillation in a periodic manner just like a pendulum or the mainspring of a watch.

We can easily view the parts of a vortex by adding a little glycerin to water. We then put the water in a clear cylinder with a hole in the bottom. The water is stirred so that a vortex funnel is formed. We then add a few drops of food coloring to the water.

The entire vortex comes alive. We can see the layers of formative surfaces as well as the rhythmic pulsation of the 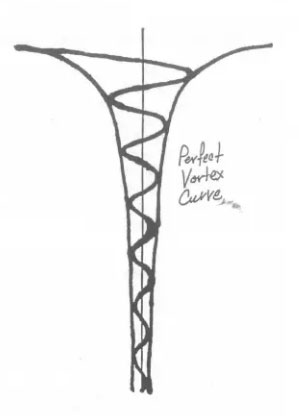whole. The inner surfaces spin more rapidly than the external ones and form cork screw like patterns that remind us of fancy sea shells.

If we look at the vortex from above we see a hole which is the suction center. We drop a small piece of wood into the water and find that the wood circles around the vortex hole, first moving slowly and then more rapidly as it approaches the center. It then circles around the vortex throat in an eccentric manner and is projected to the outside layers again. The wood is actually describing an ellipse in which the focus of the ellipse is the center of the vortex throat. On examination we discover that the water circulating around the vortex follows the exact laws of planetary motion. In fact the planets of our solar system follow the exact same circulation in their orbits around the sun. The sun is the focus of the elliptical planetary orbits. This law of planetary motion was discovered by Johannes Kepler hundreds of years ago! The vortex in its laws of movement is a miniature copy of the solar system. On a larger scale it is found in the great stellar nebulae.

Our small piece of wood eventually gets caught in the center of the vortex and is drawn to the bottom of the cylinder.

According to Dr. Schwenk, the vortex has other properties that suggest that it may have cosmic connections: If a small floating object with a fixed pointer is lowered into a vortex, it will circle around and around with the pointer always pointing in the direction which it was originally aimed. It acts just like a compass needle! It will always be directed toward some point in infinite space. According to Schwenk this shows that a vortex is always oriented as if it were held in place by invisible cosmic threads.

The vortex is a miniature model of the entire universe. Its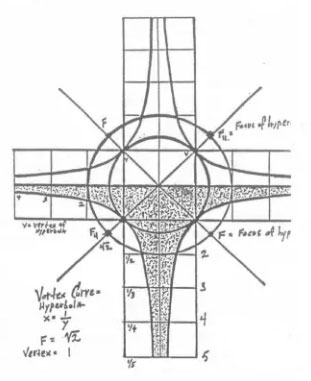 orientation in space corresponds to the fixed stars, its layered circulation corresponds to planetary motion and the suction center corresponds to the central sun.

The velocity of fluid at any point in a vortex is equal to a physical constant divided by the radius from the suction center. That is to say that velocity increases as the radius gets smaller. In a perfect vortex, as the radius approaches zero its fluid velocity will approach infinity. As infinite velocity is impossible in the physical universe, something has to give. In the case of water, the molecules begin to dissociate into a vapor. This dissociation is accompanied by the generation of high voltage electricity.

We have measured charges as high as 12,000 volts in the exact center 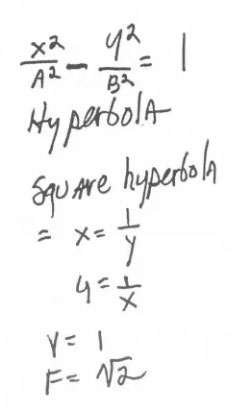of a liquid vortex! The pressure in the center of a vortex is theoretically infinitely negative. In this condition, space would be turned inside out.

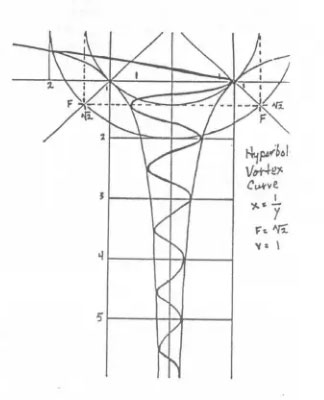
The exact shape of a vortex is a hyperboloid or hyperbola of rotation. From elementary geometry we may recall the formula of a hyperbola.

We find that the curve of a vortex is a special hyperbola which is known as a square hyperbola. In the liquid vortex if the Vertex is 1, the Focus is equal to the square root of 2.

In the diagram (above right), a square hyperbola is represented. The shaded portion represents the cross sectional form of a liquid vortex. The mirror image above the shaded portion is the imaginary hyperbolic force field above the physical vortex below. The V in the diagram is the peak of the curve, and is called the vertex. As with other conic sections such as the parabola and the ellipse the hyperbola also has focal points which are represented by F.

Viktor Schauberger, the German Forestmeister observed the liquid vortex in nature. He spent a lifetime observing the 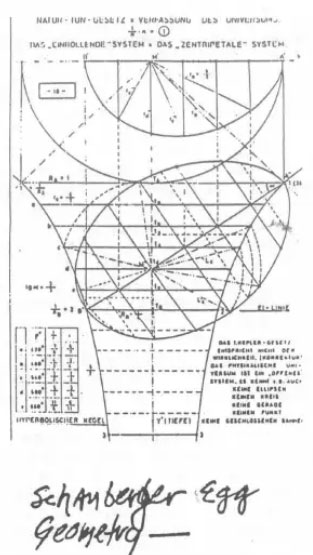flow of mountain streams in the virgin forests of Bavaria and Austria. The world is in great debt to this pioneer. He observed many phenomena associated with the liquid vortex. These phenomena include: energy discharges such as halos, and ball type lightning; levitation – in which heavy egg shaped stones float on the top of a vortex, the production of virgin water or edel wasser (living water); the purification of polluted water; and the production of free energy.

Schauberger and his son Walter who is also a pioneer in this area of investigation developed egg shaped vortex reaction chambers. These chambers are called implosion chambers as the energy developed is centripetal rather than centrifugal. He maintained that centripetal energy is the basis of life whereas centrifugal energy is the basis of decay and destruction.

In the same way that a hyperbole is a conic section, Schauberger reasoned that the perfect shape for a vortex chamber was an egg shape which is a cross section cut through the hyperboloid form of rotation developed from the square hyperbola. In other words, a cross sectional cut through the vortex throat.

The evolution of the Schauberger egg is shown in the next diagram.

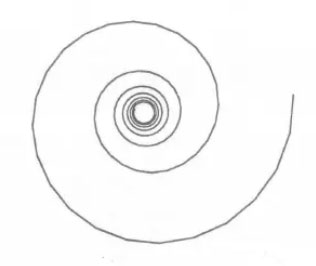
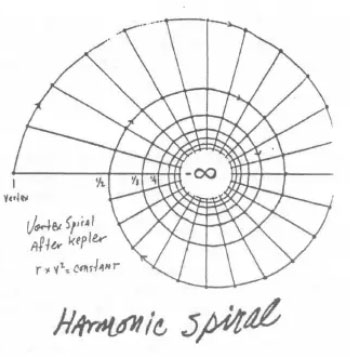The vortex spiral when viewed from above is a harmonic spiral first discovered by Kepler. This spiral is shown in the diagram. As it approaches the center from the outside, it decreases in size with each turn from unity on the outside to 1/2, 1/3, 1/4, 1/5, etc.

In our research, we have been looking at another possibility for the perfect vortex reaction chamber. If we look at the formulas for the hyperbola and the ellipse we find that they are exactly the same except for the sign in between the x and y portions.

In the case of the hyperbola, the figure is open and the ends of the lines never touch each other. In the case of the ellipse we have a closed curve which when rotated about the axis will yield a ellipsoidal container.

We reasoned that the best container for vortex would be the mathematical compliment or inverse to the hyperbola. A type of 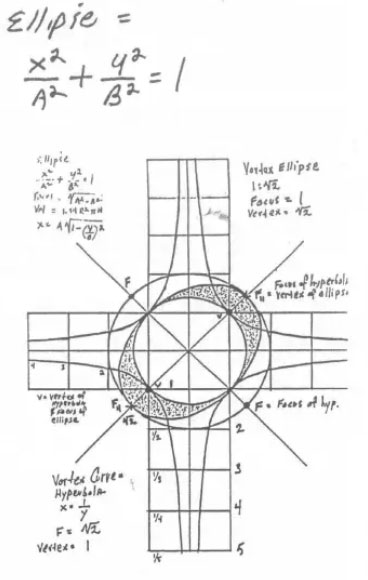ellipsoid.

The question became one of discovering the exact mathematical inverse to the the hyperbola. However, as the water vortex curve is a square hyperbola, the first thought is that the inverse is a circle which is the cross section of a sphere. This shape turned out to be a very poor container for a vortex, as a matter of fact it was the worst container generating a perfect vortex.

We went back to the drawing board and finally derived the exact inverse form. This form is an ellipse that has points that are exactly tangent to the significant points on the hyperbola. As we can see in the diagram to the right, we have an ellipse shape which is superimposed on the square hyperbola. The outline of the ellipse is shaded so that it can be easily seen.

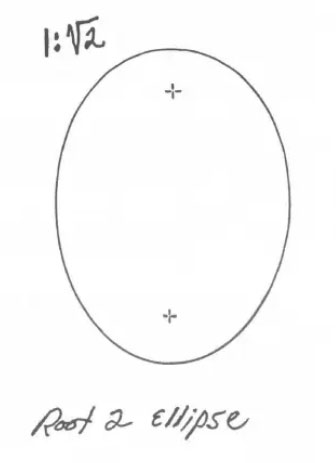This ellipse is indeed the exact compliment to the square hyperbola. The vertex of the hyperbola is the focus of the ellipse, and the focus of the hyperbola is the vertex of the ellipse. The vertexes of the diagonal hyperbolas are just touching the narrow sides of the ellipse. The length to width ratio of this ellipse is one to the square root of two. We can call this ellipse a root two ellipse.

An easy test to see which container is indeed the perfect container for a vortex is to construct various containers of equal volume and then drill small holes in the bottoms.

The holes are plugged and the containers are filled with water. The water is given a rotational momentum by stirring with a spoon. The hole is then unplugged and the formation of a vortex is observed. It will be found that different 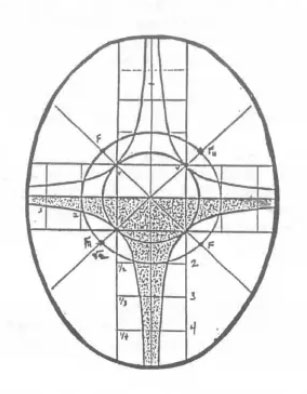shaped containers require different sized holes to sustain a vortex flow discharge by gravity alone.

When the hole is too small the water loses momentum and simply flows from the container in a solid stream which is devoid of vortex flow. When the hole is enlarged, a point will be found at which the increased rate of flow will provide enough gravitational energy to sustain a vortex.

The closer the container is to the perfect curve for sustaining a vortex, the smaller the required hole and therefore the smaller the required energy for sustaining a vortex. The perfect container will be one that develops a resonance with the hyperbolic vortex discharge. At resonance the vortex shaped flow will be sustained with minimal flow rate and therefore minimal energy.

Our ongoing research indicates that the root two ellipse is indeed the perfect shape for a vortex implosion chamber.

Archetypal Vortex

In the beginning of this paper we indicated that the vortex is a universal law of the Universe, it is the sustaining form of practically all physical phenomena. The ancient Vedic texts of India indicate that the shape of the Universe is ellipsoidal. Perhaps that is why our galaxies have vortex forms.

Dr. T.J.J. See, was Professor of Mathematics, formerly in charge of the 26 inch Equatorial Telescope of the U.S. Naval Observatory, Washington, D.C.

In 1943 he published a monumental series of 10 volumes entitled: Wave Theory! Discovery of the Cause of Gravitation. His books consisted of thousands of pages and were based on the mathematical discovery of the basis of Magnetism, Electrodynamics and other forces of nature obeying the geometrical law of the inverse squares; with complete mathematical and physical analysis based on Fourier’s Wave Theorem of 1802.

Dr. See shows that the entire physical universe revolves around the rectangular (square) hyperbola. The hyperbola referred to its asymtotes as in our vortex diagrams is the basic curve of multiple phenomena including the inverse square law of electromagnetics, the laws of magnetism, the temperature of the sun at any given point from its center outward, the surface to volume relationships of all matter, the structuring forces binding all matter, the laws of gravity, and the laws of planetary motion.

For the moment, we have all we can do in our research of the liquid vortex and its potential uses in air and water purification.

We currently use the energy phenomena associated with the liquid vortex as part of a system for making highly charged colloids. This charge which is known as the zeta potential is extremely important in colloidal behavior inside and outside of the living system.

Using the square hyperbolic curve we can show that as colloidal particles are made smaller and smaller, the surface to volume ratio goes up at a spectacular rate.

When these tiny pieces of matter are charged to high potential at a molecular level, the surface energy of these colloids act to catalyze a large number of physical processes which cannot be demonstrated without these high surface energy conditions.

We hope this brief description of the vortex will help to launch our readers into new frontiers of their own.